Shapes and generic cells

gdsfactory provides some generic parametric cells in gf.components that you can customize for your application.

Basic shapes

Rectangle

To create a simple rectangle, there are two functions:

gf.components.rectangle() can create a basic rectangle:

import gdsfactory as gf

r1 = gf.components.rectangle(size=(4.5, 2), layer=(1, 0))
r1.plot()

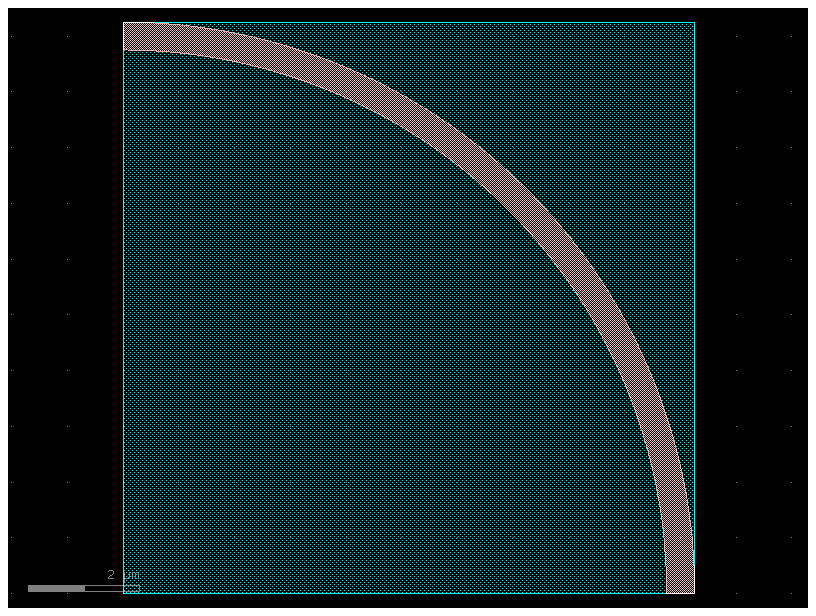
gf.components.bbox() can also create a rectangle based on a bounding box. This is useful if you want to create a rectangle which exactly surrounds a piece of existing geometry. For example, if we have an arc geometry and we want to define a box around it, we can use gf.components.bbox():

c = gf.Component()
arc = c << gf.components.bend_circular(radius=10, width=0.5, angle=90, layer=(1, 0))
arc.rotate(90)

# Draw a rectangle around the arc we created by using the arc's bounding box
rect = c << gf.components.bbox(arc, layer=(2, 0))
c.plot()

Cross

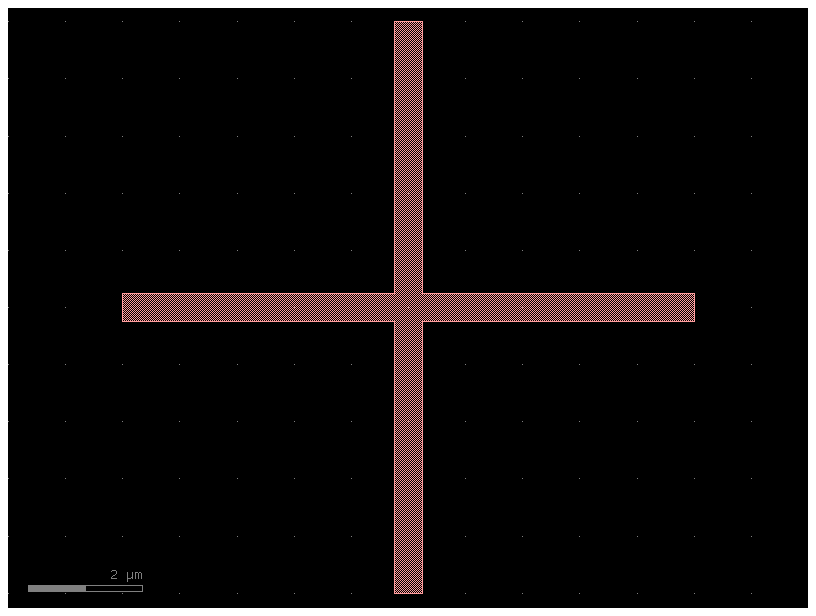
The gf.components.cross() function creates a cross structure:

c = gf.components.cross(length=10, width=0.5, layer=(1, 0))
c.plot()

Ellipse

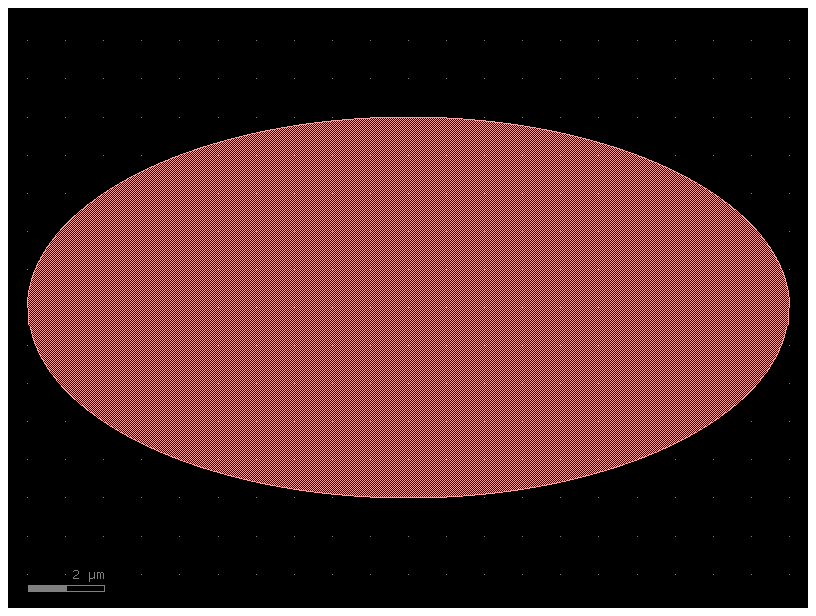
The gf.components.ellipse() function creates an ellipse by defining the major and minor radii:

c = gf.components.ellipse(radii=(10, 5), angle_resolution=2.5, layer=(1, 0))
c.plot()

Circle

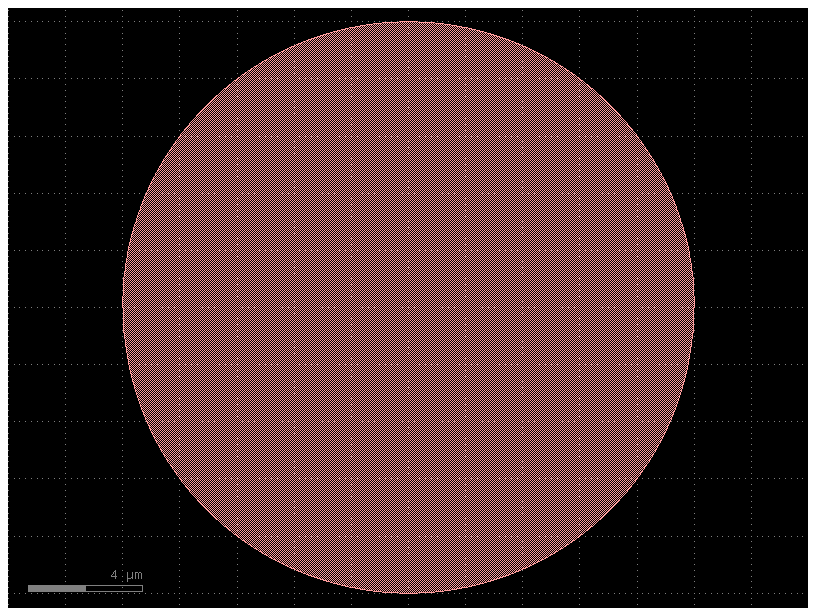
The gf.components.circle() function creates a circle:

c = gf.components.circle(radius=10, angle_resolution=2.5, layer=(1, 0))
c.plot()

Ring

The gf.components.ring() function creates a ring. The radius refers to the center radius of the ring structure (halfway between the inner and outer radius).

c = gf.components.ring(radius=5, width=0.5, angle_resolution=2.5, layer=(1, 0))
c.plot()

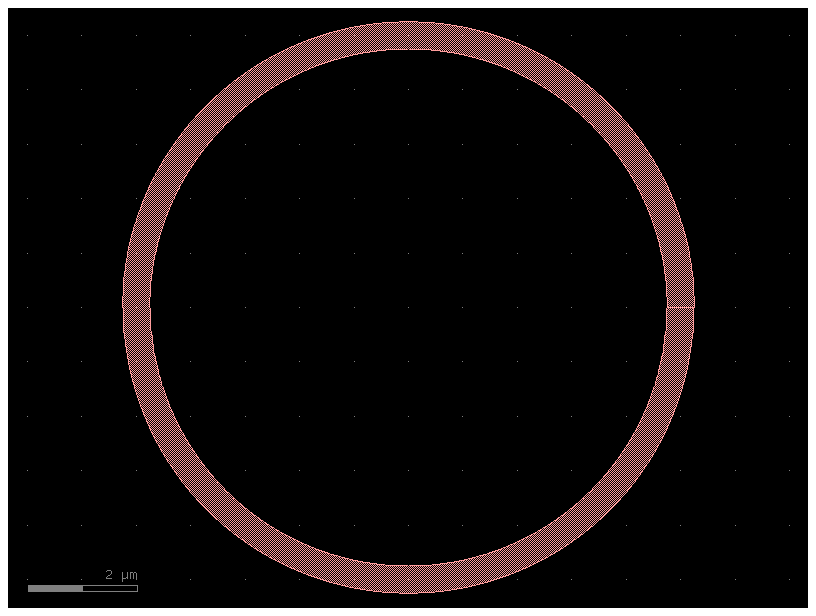
c = gf.components.ring_single(gap=0.2, radius=10, length_x=4, length_y=2)
c.plot()

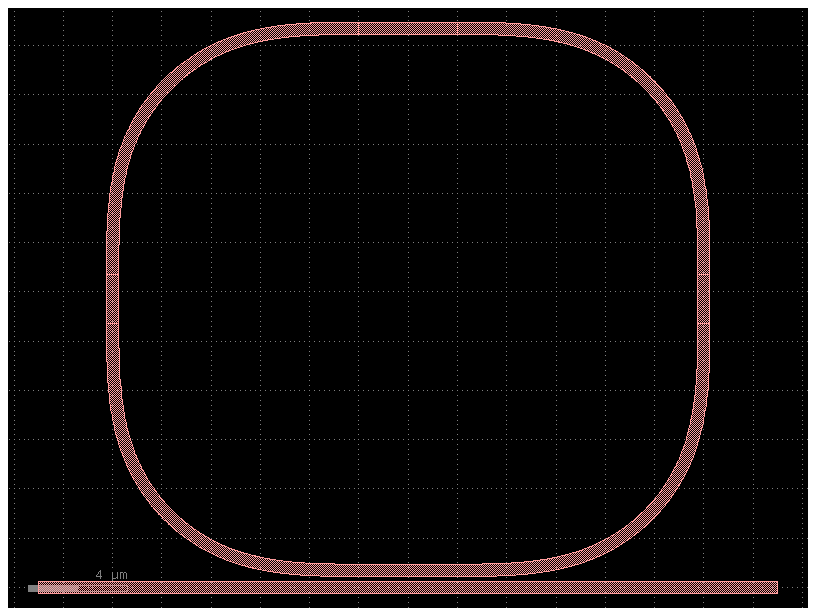
import gdsfactory as gf

c = gf.components.ring_double(gap=0.2, radius=10, length_x=4, length_y=2)
c.plot()

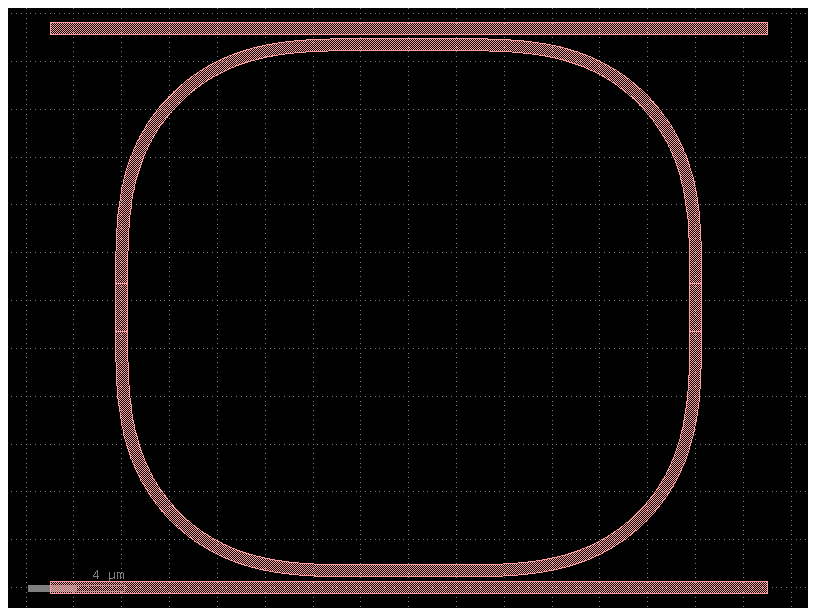
c = gf.components.ring_double(
    gap=0.2,
    radius=10,
    length_x=4,
    length_y=2,
    bend=gf.components.bend_circular,
)
c.plot()

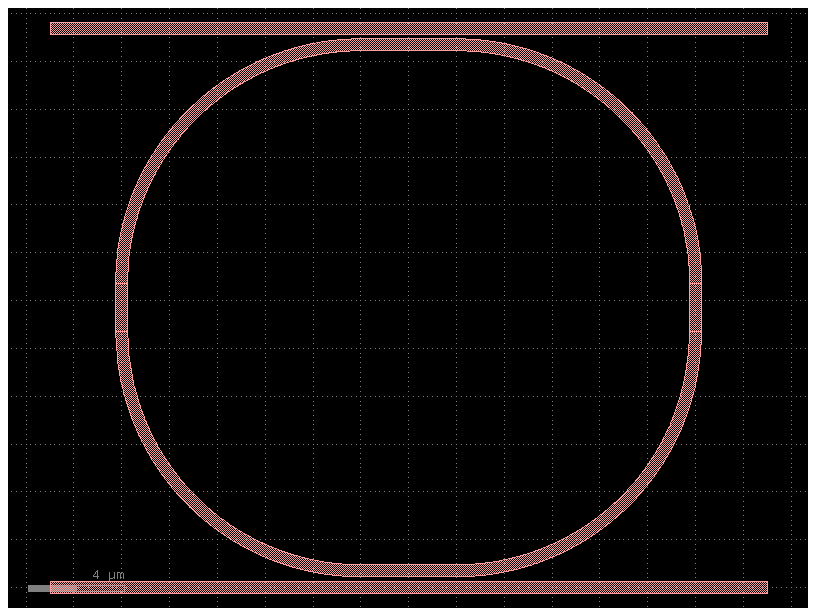
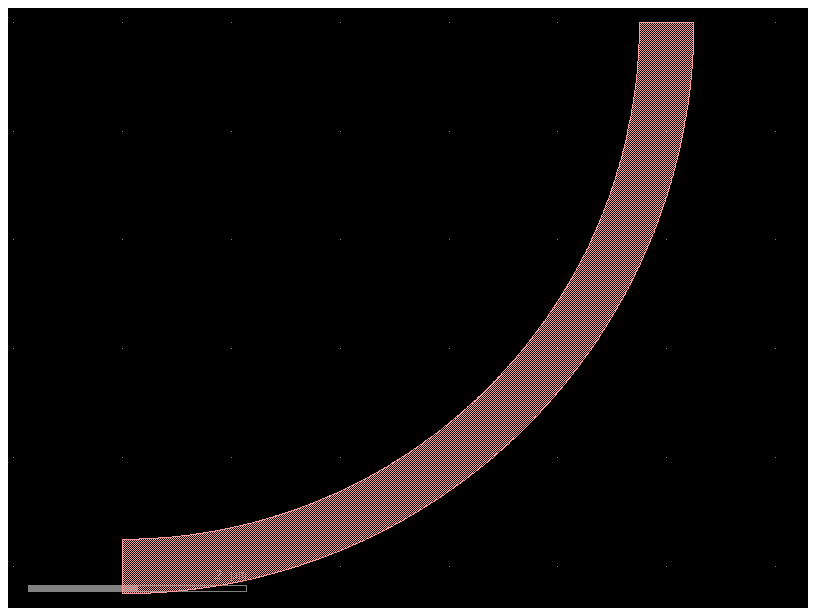
Bend circular

The gf.components.bend_circular() function creates an arc. The radius refers to the center radius of the arc (halfway between the inner and outer radius).

c = gf.components.bend_circular(
    radius=5.0, width=0.5, angle=90, npoints=720, layer=(1, 0)
)
c.plot()

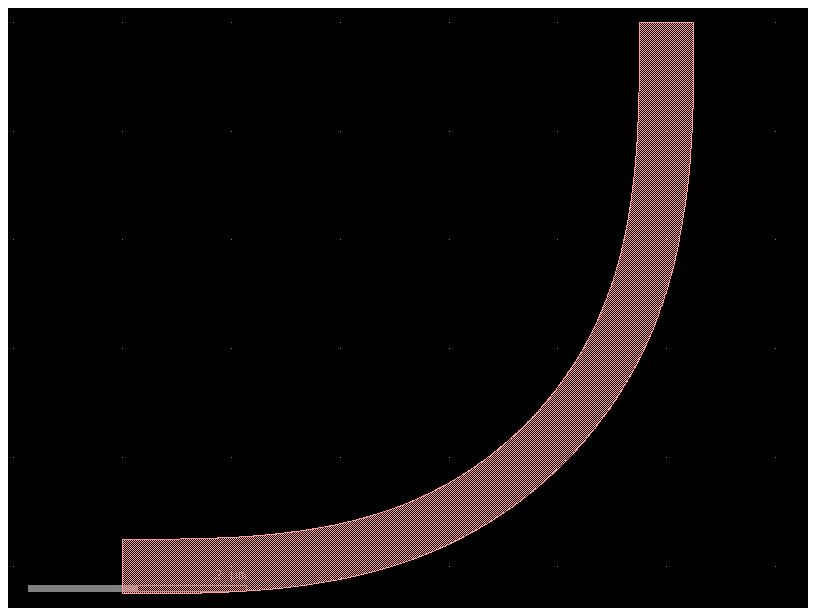
Bend euler

The gf.components.bend_euler() function creates an adiabatic bend in which the bend radius changes gradually. Euler bends have lower loss than circular bends.

c = gf.components.bend_euler(radius=5.0, width=0.5, angle=90, npoints=720, layer=(1, 0))
c.plot()

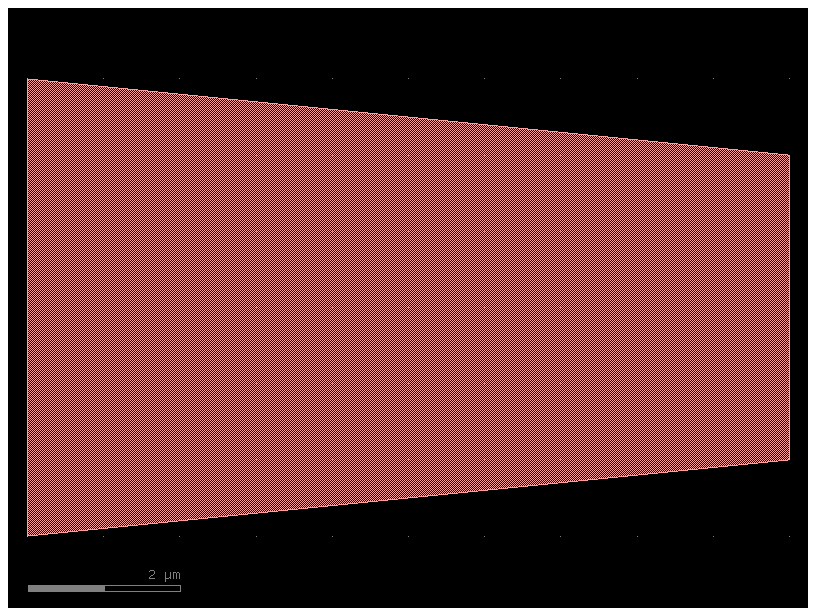
Tapers

gf.components.taper()is defined by setting its length as well as its start and end length. It has two ports, 1 and 2, on either end, allowing you to easily connect it to other structures.

c = gf.components.taper(length=10, width1=6, width2=4, port=None, layer=(1, 0))
c.plot()

gf.components.ramp() is a structure is similar to taper() except it is asymmetric. It also has two ports, 1 and 2, on either end.

c = gf.components.ramp(length=10, width1=4, width2=8, layer=(1, 0))
c.plot()

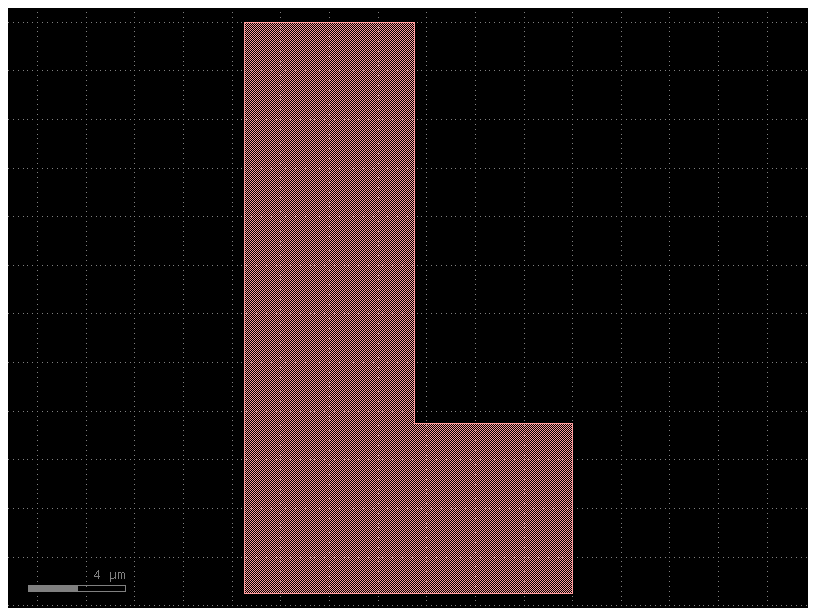
Common compound shapes

The gf.components.L() function creates a “L” shape with ports on either end named 1 and 2.

c = gf.components.L(width=7, size=(10, 20), layer=(1, 0))
c.plot()

The gf.components.C() function creates a “C” shape with ports on either end named 1 and 2.

c = gf.components.C(width=7, size=(10, 20), layer=(1, 0))
c.plot()

Text

Gdsfactory has an implementation of the DEPLOF font with the majority of english ASCII characters represented (thanks to PHIDL)

c = gf.components.text(
    text="Hello world!\nMultiline text\nLeft-justified",
    size=10,
    justify="left",
    layer=(1, 0),
)
c.plot()
# `justify` should be either 'left', 'center', or 'right'

Lithography Structures

Step-resolution

The gf.components.litho_steps() function creates a lithographic test structure that is useful for measuring the resolution of photoresist or electron-beam resists. It provides both positive-tone and negative-tone resolution tests.

c = gf.components.litho_steps(
    line_widths=(1, 2, 4, 8, 16), line_spacing=10, height=100, layer=(1, 0)
)
c.plot()

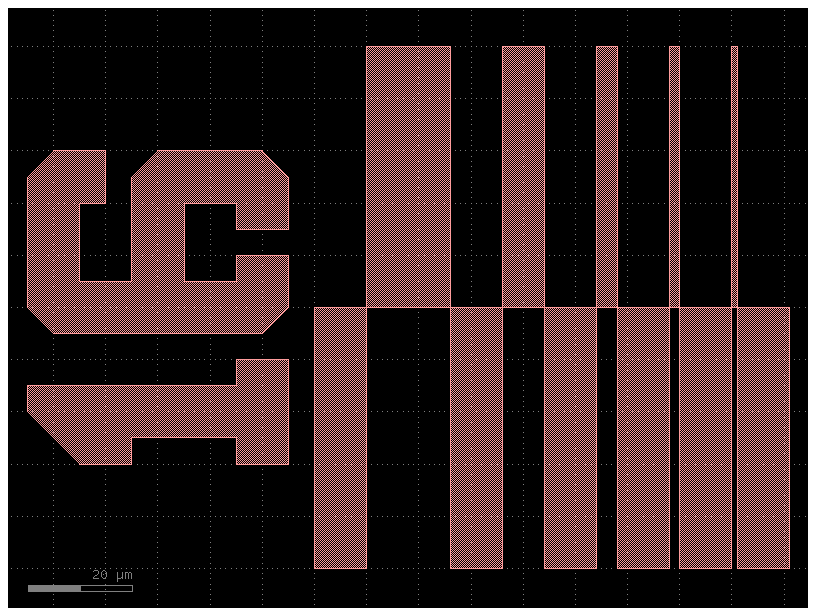
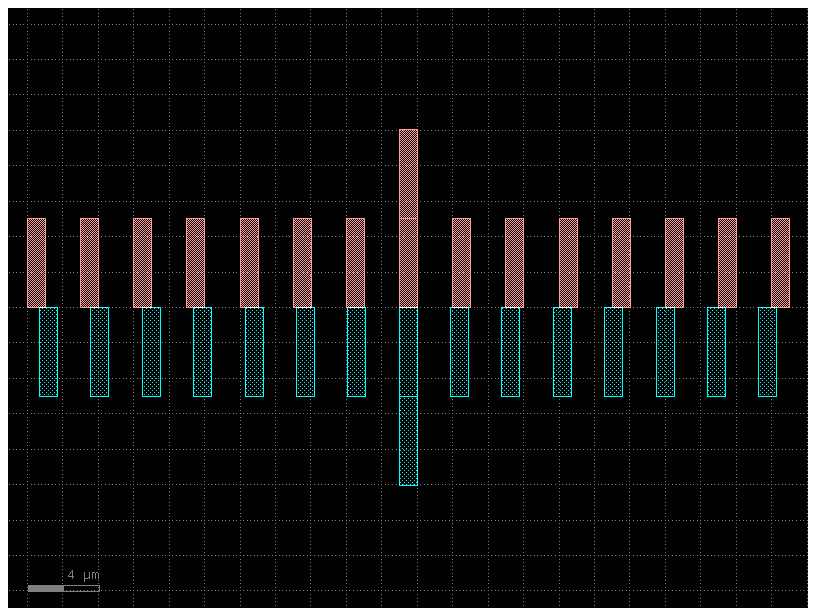
Calipers (inter-layer alignment)

The gf.components.litho_calipers() function is used to detect offsets in multilayer fabrications. It creates a set of two notches on different layers. When a fabrication error/offset occurs, it is easy to detect the magnitude of the offset because both center-notches are no longer aligned.

D = gf.components.litho_calipers(
    notch_size=(1, 5),
    notch_spacing=2,
    num_notches=7,
    offset_per_notch=0.1,
    row_spacing=0,
    layer1=(1, 0),
    layer2=(2, 0),
)
D.plot()

Paths

See Path tutorial for more details – this is just an enumeration of the available built-in Path functions

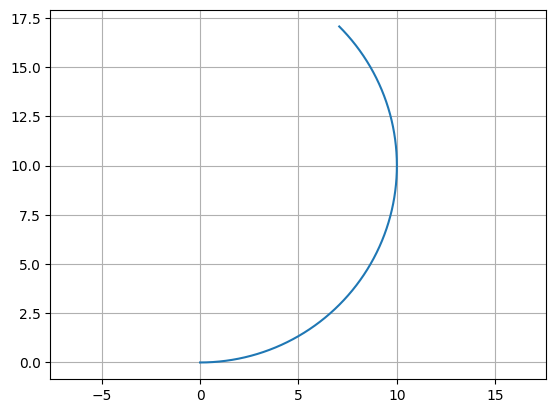
Circular arc

P = gf.path.arc(radius=10, angle=135, npoints=720)
f = P.plot()

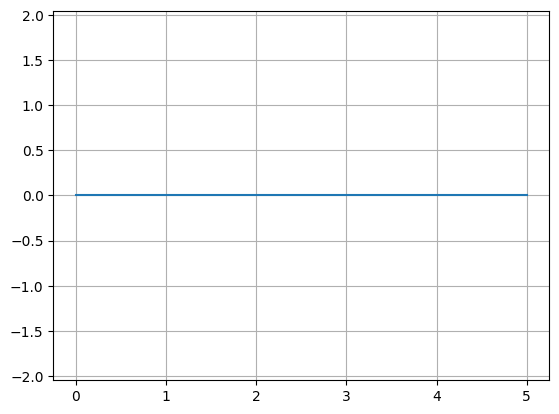
Straight

import gdsfactory as gf

P = gf.path.straight(length=5, npoints=100)
f = P.plot()

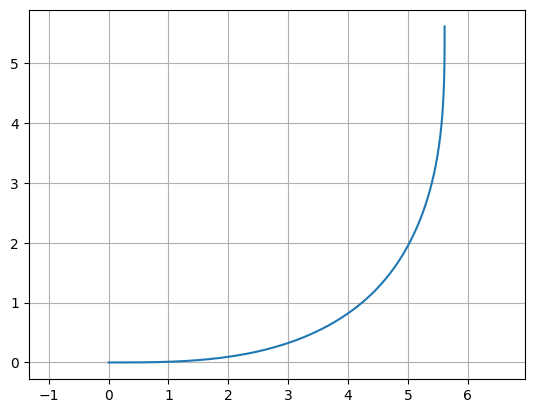
Euler curve

Also known as a straight-to-bend, clothoid, racetrack, or track transition, this path tapers adiabatically from straight to curved. Often used to minimize losses in photonic straights. If p < 1.0, it will create a “partial euler” curve as described in Vogelbacher et. al. https://dx.doi.org/10.1364/oe.27.031394.
If the use_eff argument is false, radius corresponds to minimum radius of curvature of the bend. If use_eff is true, radius corresponds to the “effective” radius of the bend– The curve will be scaled such that the endpoints match an arc with parameters radius and angle.

P = gf.path.euler(radius=3, angle=90, p=1.0, use_eff=False, npoints=720)
f = P.plot()

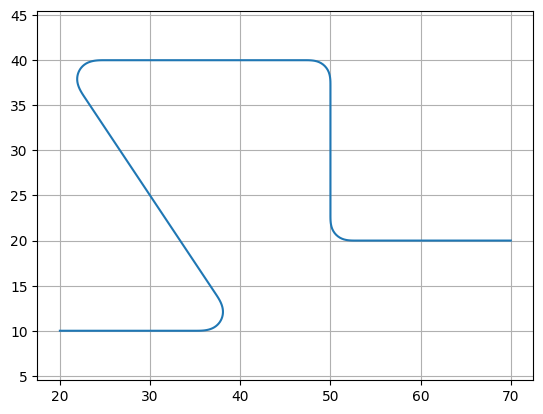
Smooth path from waypoints

import numpy as np

import gdsfactory as gf

points = np.array([(20, 10), (40, 10), (20, 40), (50, 40), (50, 20), (70, 20)])

P = gf.path.smooth(
    points=points,
    radius=2,
    bend=gf.path.euler,
    use_eff=False,
)
f = P.plot()

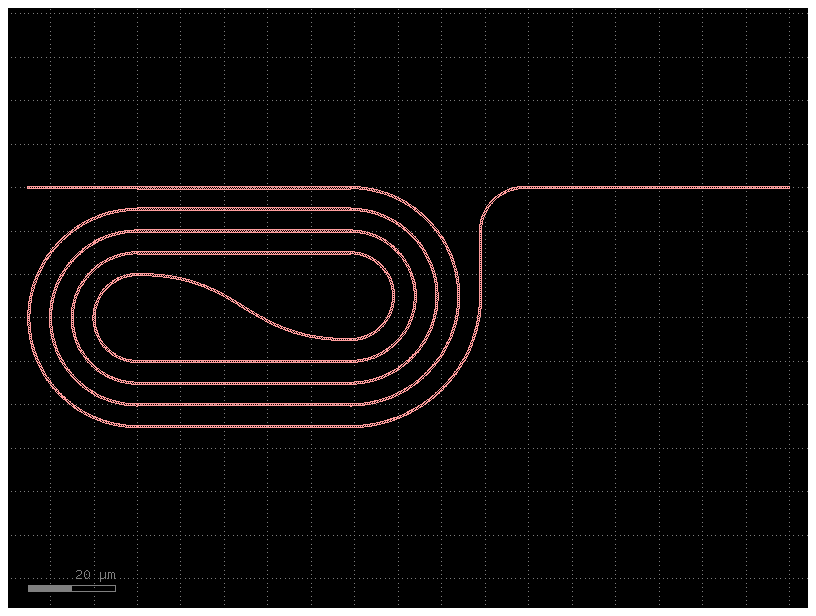
Delay spiral

c = gf.components.spiral_double()
c.plot()

c = gf.components.spiral()
c.plot()

c = gf.components.spiral_racetrack_fixed_length()
c.plot()

/home/runner/work/gdsfactory/gdsfactory/gdsfactory/components/bends/bend_circular.py:84: UserWarning: {'width': 0.5} ignored for cross_section 'strip'
  x = gf.get_cross_section(cross_section, width=width or x.width)

Useful contact pads / connectors

These functions are common shapes with ports, often used to make contact pads

c = gf.components.compass(size=(4, 2), layer=(1, 0))
c.plot()

c = gf.components.nxn(north=3, south=4, east=0, west=0)
c.plot()

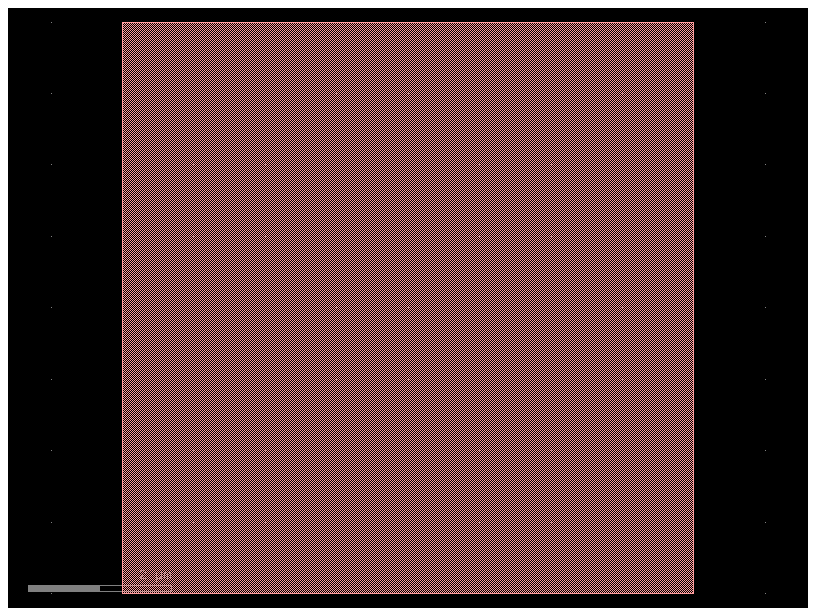
c = gf.components.pad()
c.plot()

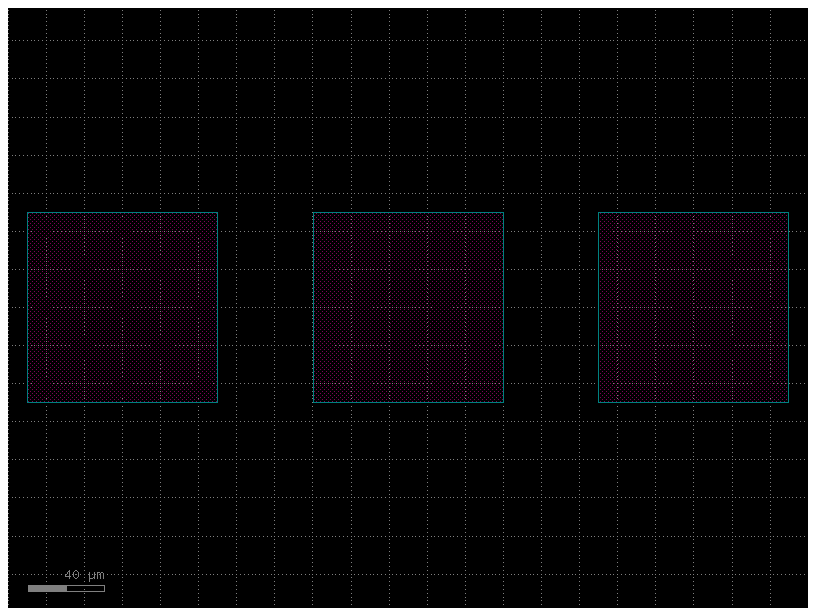
c = gf.components.pad_array90(columns=3)
c.plot()

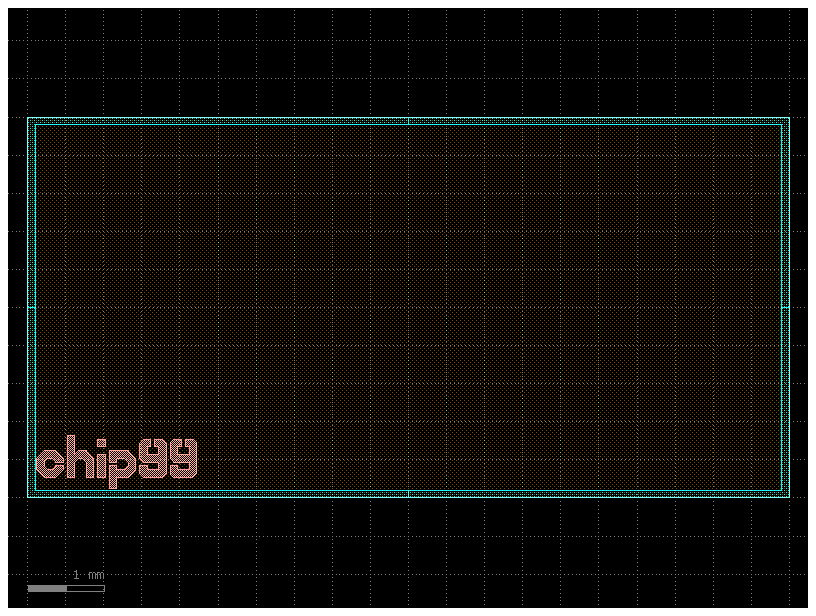
Chip / die template

import gdsfactory as gf

c = gf.components.die(
    size=(10000, 5000),  # Size of die
    street_width=100,  # Width of corner marks for die-sawing
    street_length=1000,  # Length of corner marks for die-sawing
    die_name="chip99",  # Label text
    text_size=500,  # Label text size
    text_location="SW",  # Label text compass location e.g. 'S', 'SE', 'SW'
    layer=(2, 0),
    bbox_layer=(3, 0),
)
c.plot()

Optimal superconducting curves

The following structures are meant to reduce “current crowding” in superconducting thin-film structures (such as superconducting nanowires). They are the result of conformal mapping equations derived in Clem, J. & Berggren, K. “Geometry-dependent critical currents in superconducting nanocircuits.” Phys. Rev. B 84, 1–27 (2011).

import gdsfactory as gf

c = gf.components.optimal_hairpin(
    width=0.2, pitch=0.6, length=10, turn_ratio=4, num_pts=50, layer=(2, 0)
)
c.plot()

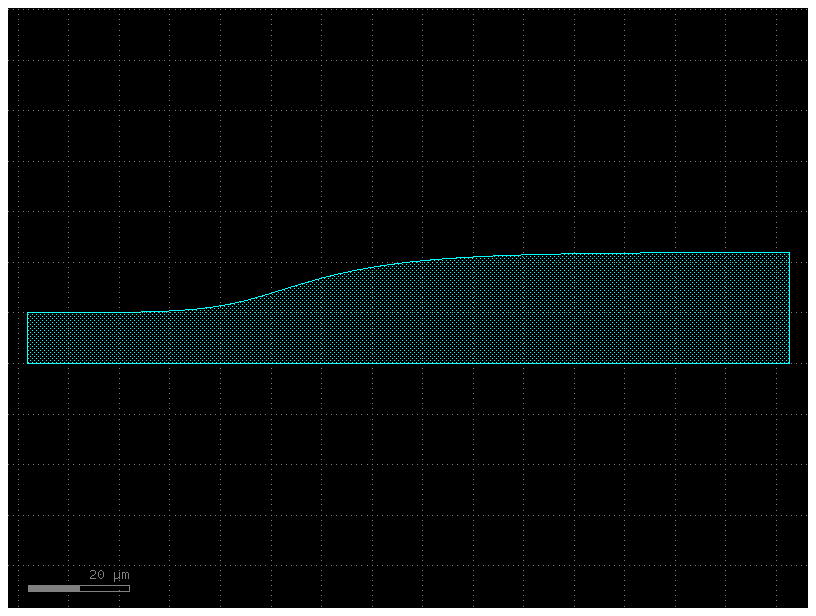
c = gf.components.optimal_step(
    start_width=10,
    end_width=22,
    num_pts=50,
    width_tol=1e-3,
    anticrowding_factor=1.2,
    symmetric=False,
    layer=(2, 0),
)
c.plot()

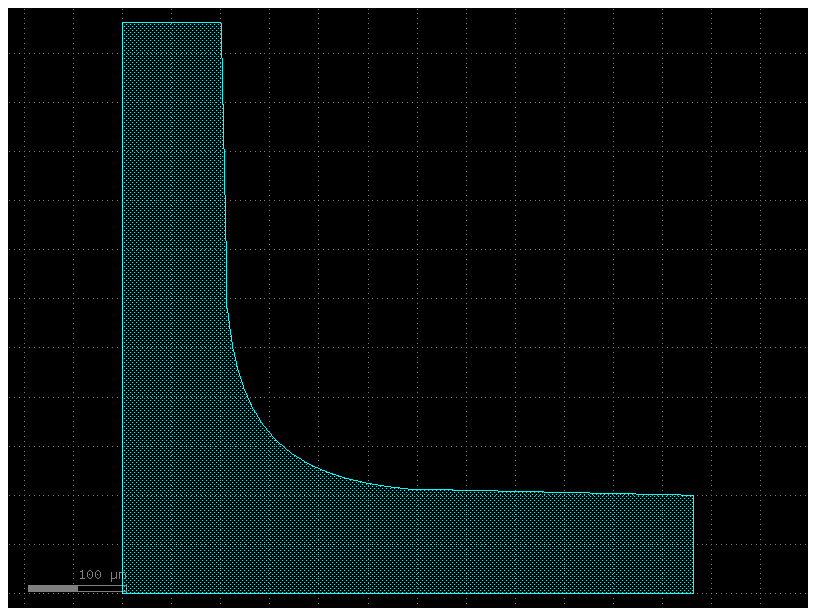
c = gf.components.optimal_90deg(width=100.0, num_pts=15, length_adjust=1, layer=(2, 0))
c.plot()

c = gf.components.snspd(
    wire_width=0.2,
    wire_pitch=0.6,
    size=(10, 8),
    num_squares=None,
    turn_ratio=4,
    terminals_same_side=False,
    layer=(2, 0),
)
c.plot()

Generic library

gdsfactory comes with a generic library that you can customize to your needs or even modify the internal code to create the components that you need.